π/8（デルトイド）より面積の小さい星状領域、できればπ/11より面積の小さい星状領域を構成してみたいのであるが、

実際問題としてデルトイドより面積の小さい星状領域をみつけることは簡単な問題ではない。

これからはすでに知られているシェーンベルグ集合、ボレリ集合を含む５種類の異なる星状集合を扱う。

Circular arc star shaped set (Schoenberg)

Asteroid arc star shaped set (Borrelli)

Peritrochoid arc star shaped set

Pentagram and N-cusped star shaped set

Slender N-cusped star shaped set

＝＝＝＝＝＝＝＝＝＝＝＝＝＝＝＝＝＝＝＝＝＝＝＝＝＝＝＝＝＝＝＝＝＝＝

ペリトロコイドは２つの円運動の合成

x=a・cos(αt) + b・cos(βt)

y=a・sin(αt) + b・sin(βt)

である。

epicycloid (a＞b, β＞0)、hypocycloid (a＞b, β＜0)に対して

動円＜固定円となったものが、ペリトロコイドである。peritrochoid (a＜b, β＜0)、its companion (a＜b, β＞0)

以下、黄色の線で描かれた芒星の面積を計算するが、Nが増すにつれて面積は大きくなり、デルトイドより小さな図形にはならないことがわかる。

＝＝＝＝＝＝＝＝＝＝＝＝＝＝＝＝＝＝＝＝＝＝＝＝＝＝＝＝＝＝＝＝＝＝＝
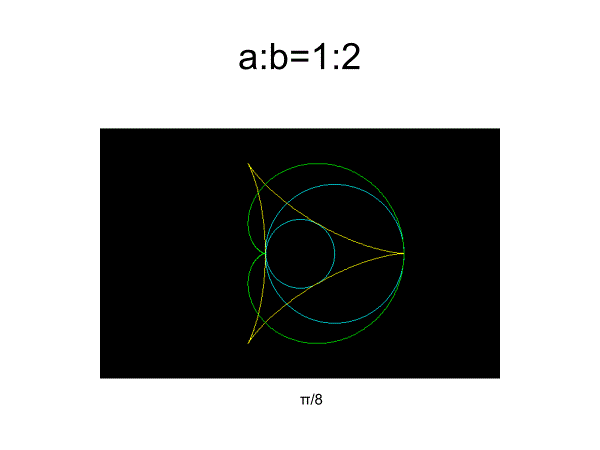
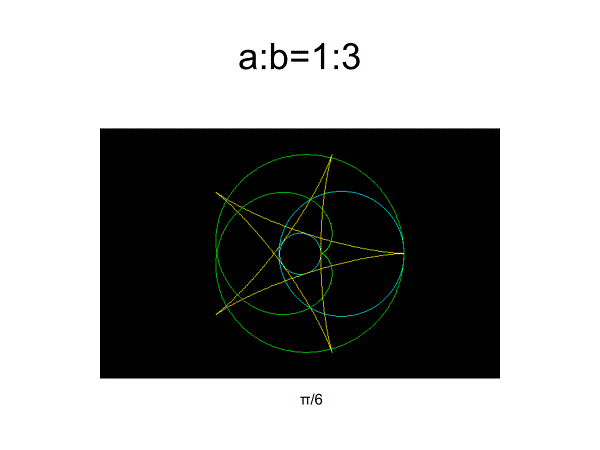
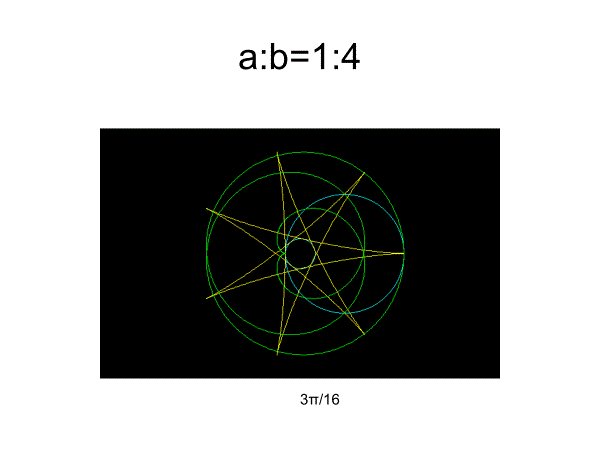
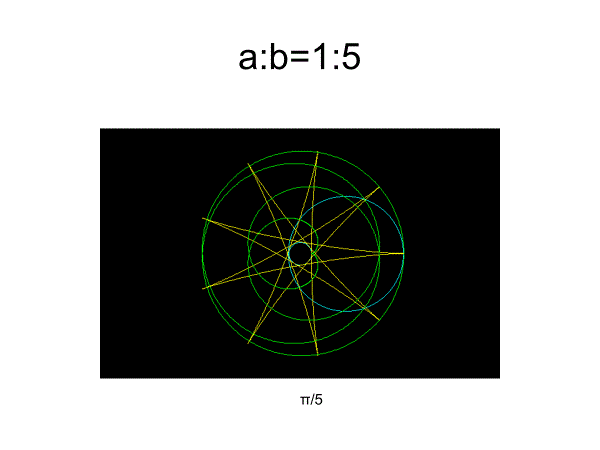