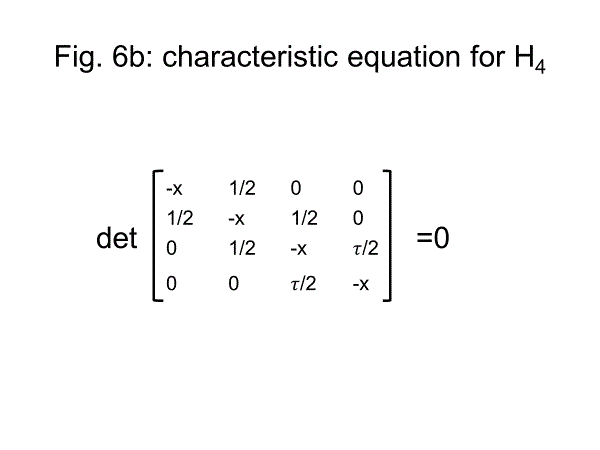モノドロミー変換の特性多項式は

Φ=Π(λ-exp(2πimi/h)

で与えられる。これは円分多項式なので因数分解される。

h=コクセター数

コクセター変換の固有値をexp(2πimi/h)として、0＜m1＜m2＜・・・＜ml＜ｈと置いたとき、これらをベキ指数と呼ぶが、

degPi=mi+1

なる関係式が成り立つ。

＝＝＝＝＝＝＝＝＝＝＝＝＝＝＝＝＝＝＝＝＝＝＝＝＝＝＝＝＝＝＝＝＝＝＝

a) A3=[3,3]

The truncated octahedron is the primitive of A3 (also non-primitive of B3) and the characteristic equation results in the second kind of Chebyshev polynomial;

U3(x)=8x3-4x=0, m={mi}={1,2,3}.

Σmi=1+2+3=6 (Antipodal distance of primitive n-parallelotope is given as n(n+1)/2.)

Π(mi+1)=2･3･4=24 ((n+1)! vertices in primitive n-parallelotope.)

Note that {mi+1}={2,3,4} reveals double, triple and quadruple rotational symmetry and Φ2(x) Φ3(x) Φ4(x) implies factorization of finite discrete space.

＝＝＝＝＝＝＝＝＝＝＝＝＝＝＝＝＝＝＝＝＝＝＝＝＝＝＝＝＝＝＝＝＝＝＝

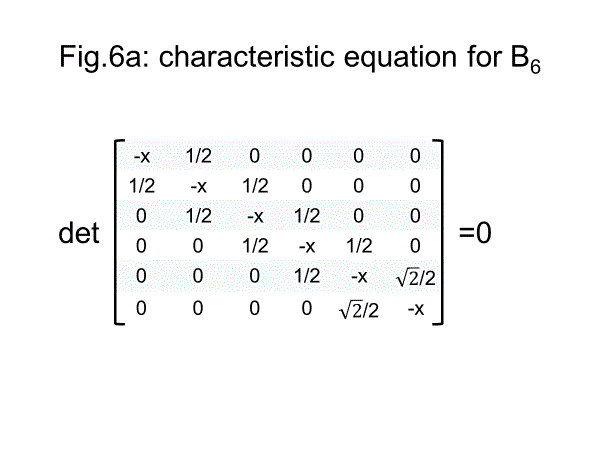
b) B6=[3,3,3,3,4]

The characteristic equation (Fig. 6a) has resulted in the first kind of Chebyshev polynomial.

T6(x)=32x6-48x4+18x2-1=0, m={mi}={1,3,5,7,9,11}. G(x)= Φ2(x) Φ4(x) Φ6(x) Φ8(x) Φ10(x) Φ12(x).

Σmi=1+3+5+7+9+11=36 (Antipodal distance is 36 and n2 in bi-submodular n-polytope.)

Π(mi+1)=2･4･6･8･10･12=46080 (=2n･n! vertices in bi-submodular n-polytope.)

Note that the number of reflecting hyperplanes and parallel-edge pairs of the primitives are n(n+1)/2 in An and n2 in Bn.

＝＝＝＝＝＝＝＝＝＝＝＝＝＝＝＝＝＝＝＝＝＝＝＝＝＝＝＝＝＝＝＝＝＝＝

c) H4=[3,3,5]

The characteristic equation (Fig. 6b) has resulted in linear combination of Chebyshev polynomials.

2τ2T4(x)-U4(x)=0, τ=(1+√5)/2, m={mi}={1,11,19,29}. G(x)= Φ2(x) Φ12(x) Φ20(x) Φ30(x).

Σmi=1+11+19+29=60, antipodal distance is 60.

Π(mi+1)=2･12･20･30=14400 vertices.

＝＝＝＝＝＝＝＝＝＝＝＝＝＝＝＝＝＝＝＝＝＝＝＝＝＝＝＝＝＝＝＝＝＝＝
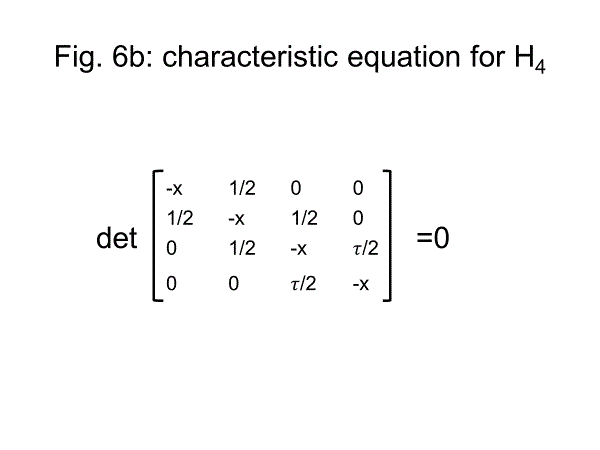