■学会にて(京大数理解析研,その205)
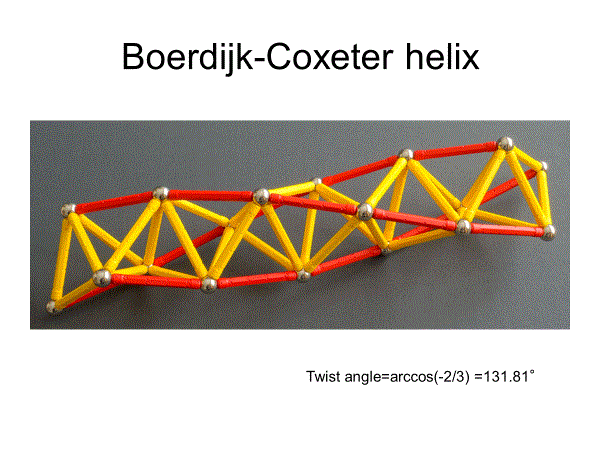
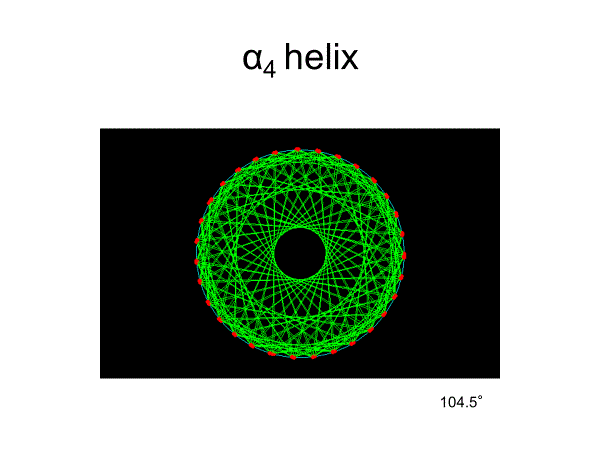
BCHの形状定数であるねじれ角はarccos(-2/3)=131.8°であり、これはπの無理数倍なので、非周期性・準結晶性を与える。すなわち、正四面体の連結数を無限に増やしてもらせん軸に沿った投影図上で頂点が重なることはない。

===================================
Twist angle ξ of α3 helix is derived from the quadratic equations, 3λ^2+4λ+3=0.
→ λ=(-2+/-√(-5))/3=cosξ+isinξ
→ cosξ=-2/3, ξ=131.81°
Because ξ/π is irrational, vertices of BCH are never overlapped in axial projection (non-periodic, quasicrystal).
===================================
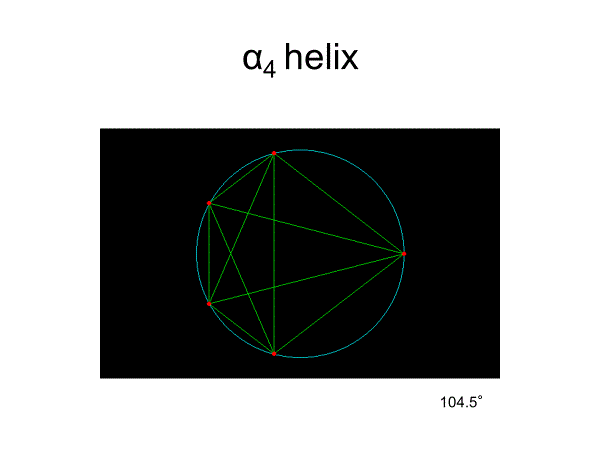
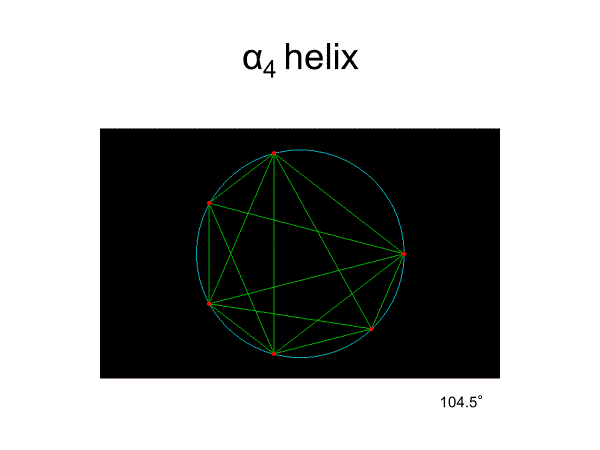
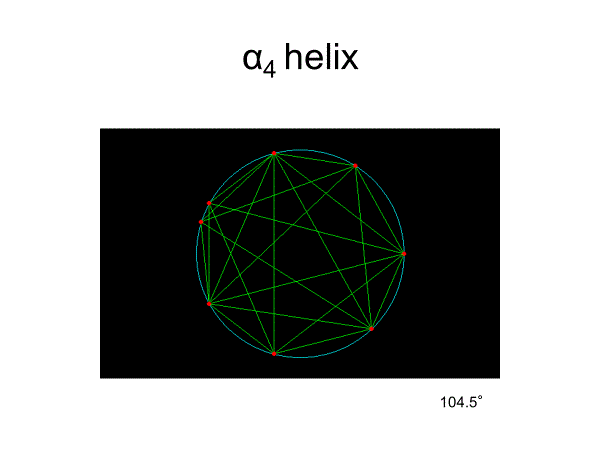
4次元の場合
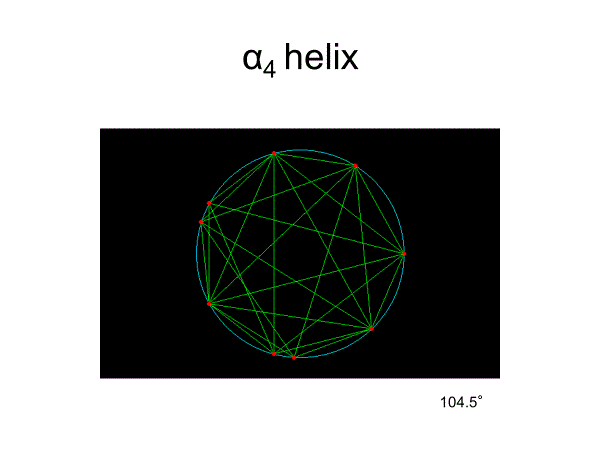
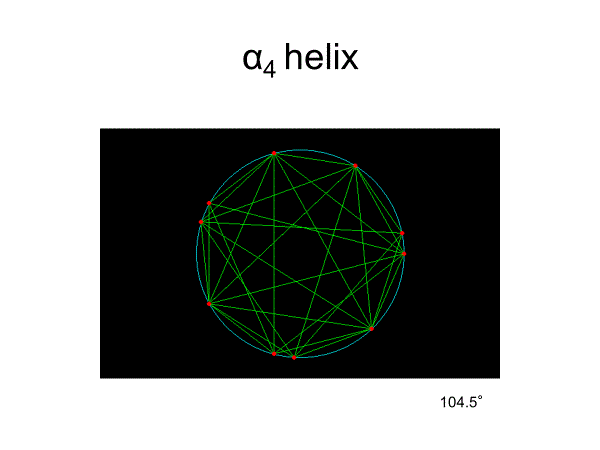
Twist angle ξ of αn helix
Twist angle ξ of αn helix is derived from the eigenvalue of following characteristic equations, as the greatest real part of roots of unity, i.e., |λi|=1, cosξ=Re[λ], sinξ=Im[λ].
4λ^3+6λ^2+6λ+4=2(λ+1) (2λ^2+λ+2)=0 (α4 helix)
→ λ=(-1±√(-7))/4=cosξ+i・sinξ
→ cosξ=-1/4, ξ=104.48°
===================================


===================================












