一般に

　　ｘ＝ｗ（ｃｏｓθ）^3−３ｓｉｎθ

　　ｙ＝ｗ（ｓｉｎθ）^3−３ｃｏｓθ

荷重ｗが大きいときアステロイドに、ｗが小さいとき円に近づくことがわかる。

＝＝＝＝＝＝＝＝＝＝＝＝＝＝＝＝＝＝＝＝＝＝＝＝＝＝＝＝＝＝＝＝＝＝＝

　　ｘ＝２（ｃｏｓθ）^3−３ｓｉｎθ

　　ｙ＝２（ｓｉｎθ）^3−３ｃｏｓθ

＝＝＝＝＝＝＝＝＝＝＝＝＝＝＝＝＝＝＝＝＝＝＝＝＝＝＝＝＝＝＝＝＝＝＝

　　ｘ＝（ｃｏｓθ）^3−３ｓｉｎθ

　　ｙ＝（ｓｉｎθ）^3−３ｃｏｓθ

　　ｘ＝３ｓｉｎθ＋ｃｏｓ^3θ

も特異点をもたずに正三角形に内接しながら回転することができる円以外の図形である。

＝＝＝＝＝＝＝＝＝＝＝＝＝＝＝＝＝＝＝＝＝＝＝＝＝＝＝＝＝＝＝＝＝＝＝

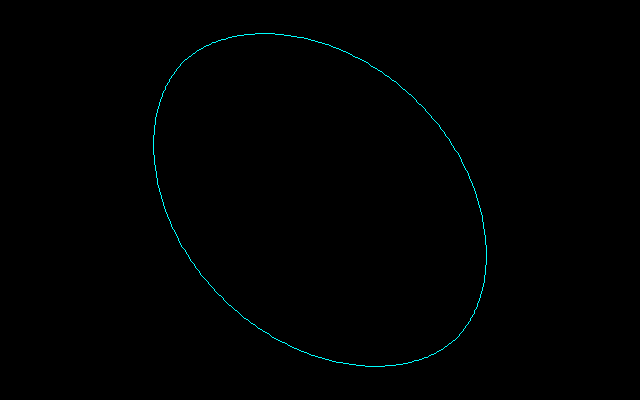
　　ｘ＝.5（ｃｏｓθ）^3−３ｓｉｎθ

　　ｙ＝.5（ｓｉｎθ）^3−３ｃｏｓθ

＝＝＝＝＝＝＝＝＝＝＝＝＝＝＝＝＝＝＝＝＝＝＝＝＝＝＝＝＝＝＝＝＝＝＝

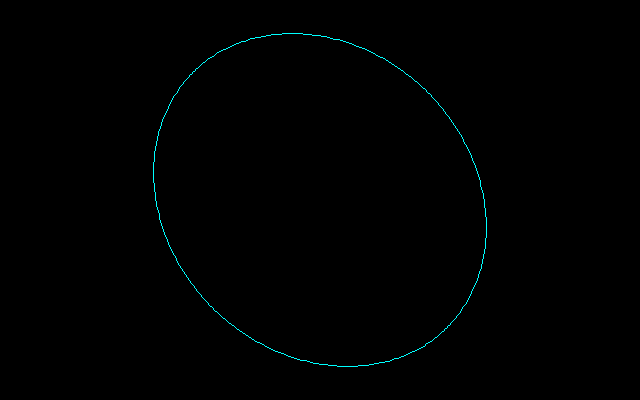
　　ｘ＝.1（ｃｏｓθ）^3−３ｓｉｎθ

　　ｙ＝.1（ｓｉｎθ）^3−３ｃｏｓθ

＝＝＝＝＝＝＝＝＝＝＝＝＝＝＝＝＝＝＝＝＝＝＝＝＝＝＝＝＝＝＝＝＝＝＝
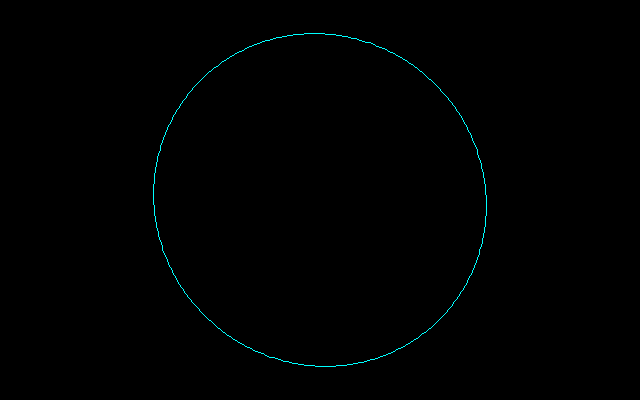